Part I:
Review of asymptotes via Asymptote Bingo
Part II:
Introduction to rational functions via this foldable:
*I think you could use this in an Interactive Notebook if you just deleted Example 3.
Part III:
Part III:
Exploring rational functions via Desmos
This was my favorite. Oh, Desmos, how I love thee. I wrote this literacy/technology activity for my students and then we headed to the Math Lab together to work on the computers:
We have really nice, big screens in the Math Lab so the kids were able to get beautiful and clear pictures of these functions, which (I think) a typical handheld graphing calculator can't quite provide. Here's what I loved: The kids would graph the function in question, for example this:
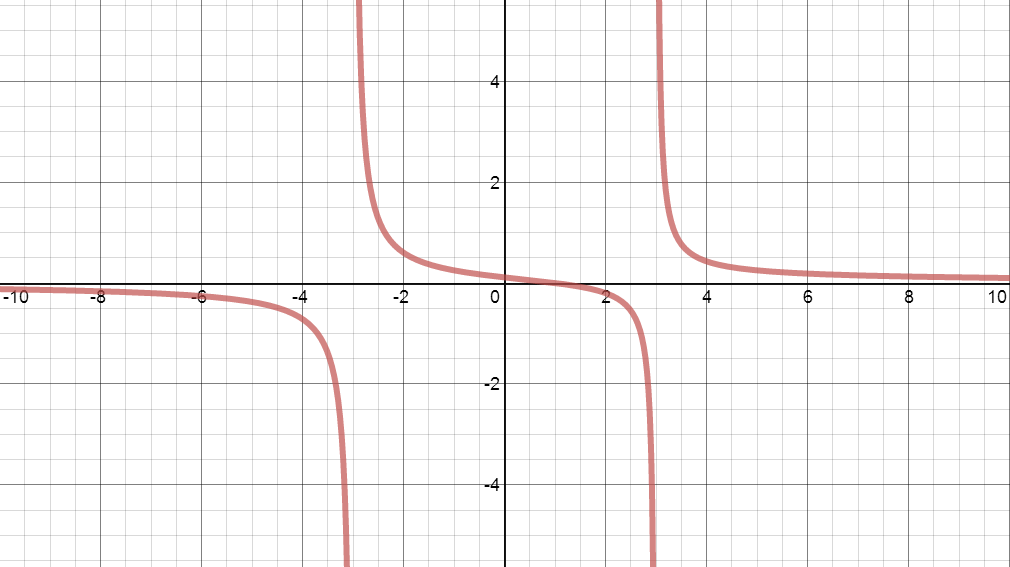
And then they were asked to analyze. I asked them to graph all their asymptotes and highlight all intercepts. So, if they accidentally said that the horizontal asymptote was x=0, when they graphed their answer, they (usually) immediately identified their mistake and made the appropriate corrections. (Or, at the very least, they raised their hands and told me, "This doesn't look right to me...") If done correctly, their ending picture should have looked something like:
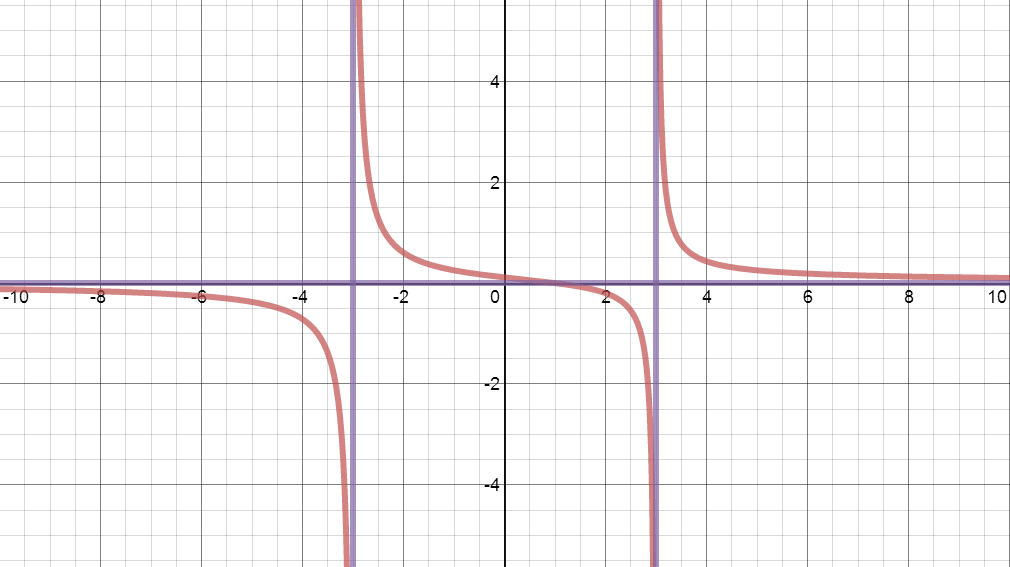
So beautiful and clean!
Part IV:
Solving rational equations through graphing and technology
Including review and assessment, I spent just over a week on this unit (like I said, it was fast). But I'm pretty happy with it--especially our day in the Math Lab. I worked out some issues with the activity, so I'm interested to use it again (I want to try it in PreCalculus) and see how it goes the second time around.
Part IV:
Solving rational equations through graphing and technology
Including review and assessment, I spent just over a week on this unit (like I said, it was fast). But I'm pretty happy with it--especially our day in the Math Lab. I worked out some issues with the activity, so I'm interested to use it again (I want to try it in PreCalculus) and see how it goes the second time around.
Thanks for sharing. I added a link to your post here
ReplyDeletehttp://msmathwiki.pbworks.com/w/page/57743234/Algebra%202%20INB#view=edit
@aanthonya
Could you tell me what the acronyms mean on the foldable?
ReplyDeleteHi, Brooke--
DeleteIt's an acronym to help students remember that degrees of the numerator/denominator determine horizontal asymptotes:
BOBO: biggest on bottom: [y=]0
BOTN: biggest on top: none
EATSDC: exponents are the same: divide coefficients
Sounds like a bad sci fi movie, but kids do seem to remember it :)
Awesome! Thank you so much! Do you have the foldable in doc form? Scribd isn't letting me download it. How did you have them fold it? I have been using INB's this year and have made a ton of awesome foldables and activities that really help kids.
ReplyDeleteSure thing! Would you mind emailing me at Rebecka dot Peterson at gmail dot com? And I'll send it your way :)
DeleteDid you get my e-mail? Wanted to make sure I sent it to the right account.
DeleteThank you for this information, hope to read more informative essay writing services here.
ReplyDeleteI was search for this information but there are extremely incomplete assets. Thank you for given that this information…
ReplyDeleteI really like your modern stylish ideas which you have shared about manipulative. This blog provide extraordinary and exact
ReplyDeletelook at my profile
information for which I was searching.
I just like this book and I guess this place on book is awesome and it is practically precisely the way that Trish composed it. Its like here http://www.bang2write.com/2015/10/5-habits-of-highly-productive-writers-by-julie-ellis.html I am anticipating her next book and I am so happy it's practically prepared.
ReplyDeleteGreat blog and post thanks for http://awriter.org/bestessaytipscom/
ReplyDeletesharing remarkable and knowledge with us.
This web site certainly has all of the information and facts I needed about this subject and didn’t know who to ask.
ReplyDeletecasio